You might be shocked to master that you just cannot comb the hairs flat on a coconut devoid of making a cowlick. Most likely even far more astonishing, this silly assert with an even sillier title, “the bushy ball theorem,” is a happy discovery from a department of math termed topology. Juvenile humor aside, the theorem has significantly-reaching outcomes in meteorology, radio transmission and nuclear electrical power.

Right here, “cowlick” can signify both a bald location or a tuft of hair sticking straight up, like the a single the character Alfalfa sporting activities in The Very little Rascals. Of training course, mathematicians never refer to coconuts or cowlicks in their framing of the dilemma. In far more specialized language, assume of the coconut as a sphere and the hairs as vectors. A vector, often depicted as an arrow, is just something with a magnitude (or duration) and a way. Combing the hair flat in opposition to the sides of the coconut would variety the equal of tangent vectors—those that touch the sphere at specifically a single position along their length. Also, we want a sleek comb, so we really don’t enable the hair to be parted everywhere. In other terms, the arrangement of vectors on the sphere have to be constant, meaning that nearby hairs really should change way only progressively, not sharply. If we stitch these requirements with each other, the theorem claims that any way you check out to assign vectors to every position on a sphere, a thing ugly is sure to materialize: there will be a discontinuity (a component), a vector with zero duration (a bald location) or a vector that fails to be tangent to the sphere (Alfalfa). In full jargon: a continual nonvanishing tangent vector area on a sphere can’t exist.

&#13&#13

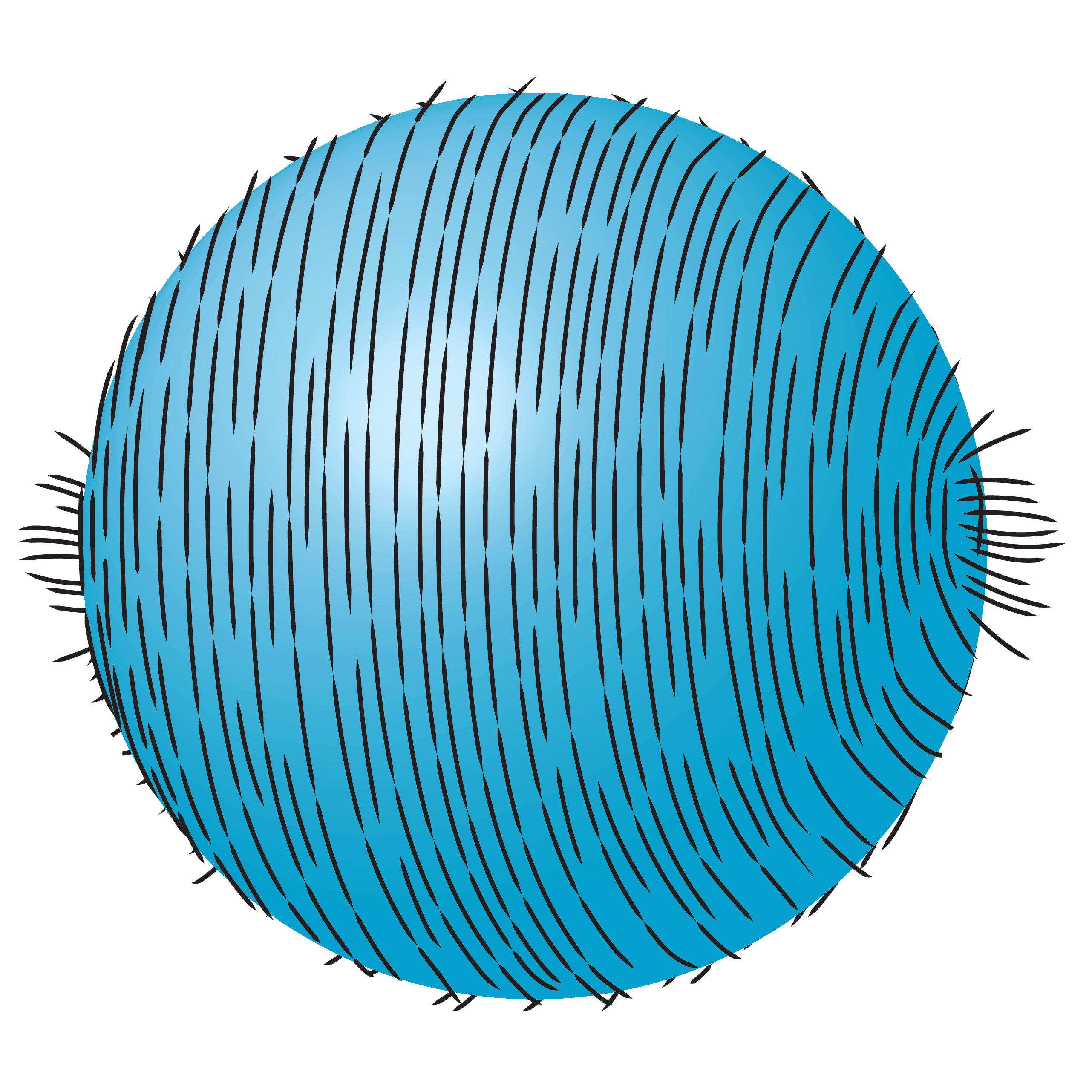
This assert extends to all types of furry figures. In the industry of topology, mathematicians research shapes, as they would in geometry, but they think about these shapes are built from an at any time elastic rubber. Although that rubber is capable of molding into other types, it is incapable of tearing, fusing or passing as a result of alone. If one form can be easily deformed into another without having doing these things, then those people styles are equal, as much as topologists are anxious. This usually means that the bushy ball theorem instantly applies to furry cubes, furry stuffed animals and hairy baseball bats, which are all topologically equivalent to spheres. (You could mold them all from a ball of Participate in-Doh with out violating the rubbery policies.)

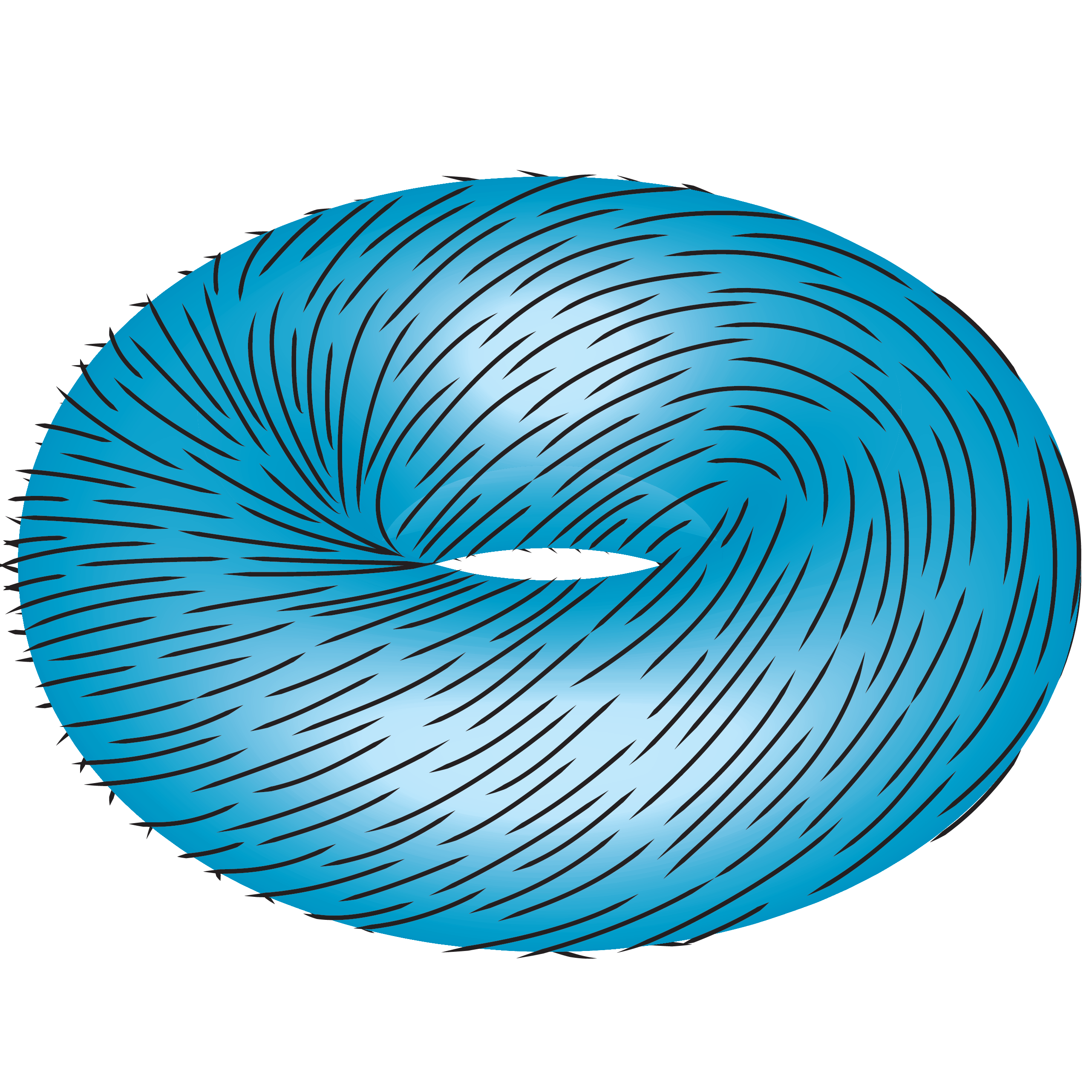
Anything that is not equivalent to a sphere is your scalp. A scalp on its very own can be flattened into a area and combed in one way like the fibers on a shag carpet. So regrettably, math can not excuse your bedhead. Doughnuts are also distinctive from spheres, so a bushy doughnut—an unappetizing impression, no doubt—can be combed easily.

&#13&#13

Here’s a curious consequence of the hairy ball theorem: there will always be at minimum a single stage on Earth where the wind is not blowing throughout the surface. The wind flows in a constant circulation all over the world, and its direction and magnitude at every single spot on the area can be modeled by vectors tangent to the world. (Vector magnitudes really don’t will need to depict actual physical lengths, such as people of hairs.) This meets the premises of the theorem, which implies that the gusts must die someplace (producing a cowlick). A cowlick could take place in the eye of a cyclone or eddy, or it could take place for the reason that the wind blows straight up towards the sky. This neat on the internet device depicts up-to-date wind currents on Earth, and you can clearly place the swirly cowlicks.

To notice one more weird ramification of the theorem, spin a basketball any which way you want. There will always be a level on the surface area that has zero velocity. Yet again, we affiliate a tangent vector with every issue centered on the way and velocity at that issue on the ball. Spinning is a constant motion, so the bushy ball theorem applies and assures a place with no velocity at all. On further more reflection, this could possibly seem clear. A spinning ball rotates about an invisible axis, and the factors on possibly conclusion of that axis never shift. What if we bored a small hole by the ball just alongside that axis to get rid of the stationary details? It looks then that every single issue would be shifting. Does this violate the furry ball theorem? No, since drilling a gap remodeled the ball into a doughnut! Even doughnuts with unusually extended, narrow holes flout the principles of the theorem—contradiction averted.

Relocating on from toy scenarios—the furry ball theorem basically imposes tangible limits on radio engineers. Antennas broadcast radio waves in distinct directions relying on design and style alternatives. Some concentrate on their signals in a specific way, although other people beam much more broadly. One may be tempted to simplify issues and build only antennas that mail equivalent-energy signals in each and every way at at the time, which are termed isotropic antennas. There’s just just one challenge: a particular hirsute fact from topology mandates that isotropic antennas just cannot exist. Photograph an orb of waves emanating from a central supply. Adequately far absent from the supply, radio waves show an electric industry perpendicular to the way they’re traveling, that means the subject is tangent to the sphere of waves. The hairy ball theorem insists that this subject ought to drop to zero somewhere, which indicates a disturbance in the antenna’s signal. Isotropic antennas provide simply as theoretical beliefs versus which we review serious antenna overall performance. Apparently, seem transmits a diverse form of wave without the need of the perpendicular assets of radio waves, so loudspeakers that emanate equal-intensity sound in each direction are doable.

Maybe the coolest application of the hairy ball theorem fears nuclear fusion electricity. Fusion ability carries enormous guarantee to—maybe sometime—help relieve the strength crisis. It has the probable to produce vast quantities of electricity without the need of the environmental fears that plague fossil fuels and with significantly less of the radioactive challenges associated with common nuclear fission reactors. In a nutshell, fusion reactors start out by getting a gas these as hydrogen and subjecting it to intense heat and stress, which rips it into its constituent pieces to type plasma. Plasma is a cloud of electrons and other billed particles that bop all around and once in a while fuse collectively to type new particles, releasing strength in the method.

There is a basic engineering hurdle when creating fusion reactors: How do you contain plasma which is 10 instances hotter than the sun’s core? No product can stand up to that temperature with out disintegrating into plasma by itself. So scientists have devised a intelligent resolution: they exploit plasma’s magnetic properties to confine it within a potent magnetic discipline. The most natural container layouts (consider packing containers or canisters) are all topologically equal to spheres. A magnetic industry around any of these constructions would sort a constant tangent vector discipline, and at this level we know what befalls this kind of hairy constructions. A zero in the magnetic discipline implies a leak in the container, which spells catastrophe for the entire reactor. This is why the main style and design for fusion reactors, the tokamak, has a doughnut-shaped chamber. The Worldwide Thermonuclear Experimental Reactor (ITER) megaproject plans to end design of a new tokamak in France by 2025, and all those concerned declare their magnetic confinement program will be “the biggest and most built-in superconducting magnet process ever built.” That is topology actively playing its portion in our clean electricity long run.

This is an viewpoint and analysis posting, and the views expressed by the writer or authors are not automatically people of Scientific American.